Introduction: It is often necessary in experimental research to quantify the dividing capabilities of cells when investigating manipulations of the cells or their environment. The growth of cell populations can be modelled by assuming each cell divides into two, and the rate of growth depends on the length of the cell cycle. However, the simplicity of this idea may lead to misinterpretations in some circumstances. The growth rate of a population may be reduced if the length of the cell cycle increases. However, the growth rate may also be reduced if only a fraction of the cells are dividing.

Importance: Using equations to model growing populations of cells requires knowledge about the cells themselves. This is particularly important when modelling the growth of cancerous cells. Many cells die quickly, leaving only a fraction of cancerous cells dividing.

Questions: How does the growth of a cell population change if only a fraction of the cells are dividing?

Methods: When modelling the growth of a population of cells, it is commonly useful to assume that every individual cell doubles with every cell cycle, i.e. the daughter cells themselves divide upon completion of the next cell cycle. This is typically expressed as an exponentially or geometrically growing population. The proliferation of cells can be quantified by the equation

where N(t) is the number of cells at time t, N₀ is the initial number of cells, and f is the frequency of cell cycles per unit time. For example if a cell completes the cell cycle two times a day, then f=2 cell cycles per day. Similarly we can write the duration of the cell cycle as 1/f, or number of days per cell cycle. The doubling time can also be calculated as 1/f. If f=2, then the duration of the cell cycle, or doubling time of a single cell, is 1/2 day.

We can graph the number of cells (N) over time (t) by choosing an initial population size, N₀. By varying f, we can see how the rate of growth affects the growth of the population.

Interpretation: As the freqency of cell cycles per unit time (f) increases, the number of cells in the population increases rapidly. For a smaller value of f, one might interpret that the cell cycle is longer (1/f is larger) and cells are not dividing as rapidly.

Alternatively, it may be the result of a reduction in the fraction of cells that are dividing. Since the exponential equation assumes all cells divide, we may be misinterpreting the graphs by assuming a reduction in the length of the cell cycle. We need a different type of equation to represent the fraction of daughter cells dividing.

Methods: The exponential equation assumes all cells divide. In some cell populations, however, only a fraction of the cells are dividing. When comparing populations, it may be feasible to assume the frequency of cells cycles per unit time (f) is similar, but the fraction of cells dividing is different. We can derive an equation that accounts for the fraction of daughter cells that divide (F).

The number of cells after one cell cycle (N₁) is the number that do not divide plus twice the number that do:

where 1-F is the fraction that do not divide and N₀ is the number of cells initially.

After two cell cycles, the number of cells (N₂) is the number of cells in the initial population (N₀) that do not divide plus the number of new cells (2FN₀) that do not divide, plus twice the number of new cells that do divide:

After several cell cycles, we can model cell population growth more generally as

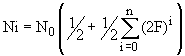
where i = 0...n is the number of cell cycles that have occurred (frequency of cell cycles per unit time (f) x time of experiment (t)).

Experimentally, the fraction of dividing cells (F) can be estimated by graphing data for a growing population of cells. Using the derived equation, we can graph the number of cells (N) over time to examine how changes in the fraction of cells dividing (F) affect the growth of the population. We will use f = 2.45 (red lines) and f = 1.88 (blue lines) cell cycles per day, as in the above graph.

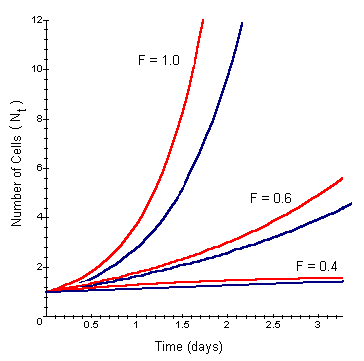
Interpretation: Each pair of lines represents either f = 2.45 (upper of each pair) or f = 1.88 (lower of each pair). Notice as we increase the fraction of cells dividing (F), the growth of the cell population increases dramatically. When F=1, the population simply grows exponentially as in our original graphs. As the fraction of cells dividing decreases, the growth of the population slows. Notice that both the fraction of cells dividing and the length of the cell cycle can affect the growth of the cell population.

Conclusions: It is important when modelling the growth of cells to apply knowledge about the cell population. A reduction in cell population growth may be attributed to an increase in the length of the cell cycle OR a decrease in the fraction of cells dividing. In many cases, it will be a combination of the two (as well as other factors) and consideration of the cell population is required to interpret the growth.

1. Look at the graph of exponential growth and estimate the doubling time for f = 2.45 and f = 1.88. Now estimate the doubling times when only 60% of the cells are dividing.

2. You experimentally estimate the doubling time of two cell populations to be 1.3 days and 2.6 days, respectively. Assuming 100% of the cells are dividing, calculate the frequency of cell cycles per day for each population.

3. Upon closer examination of the cells, you realize the frequency of cell cycles is the same for both populations, f = 0.7692 cycles per day, but only a fraction of the cells in each population are dividing. For the first population, it takes 1 cell cycle (n = tf = 1.3 days * 0.7692 cell cycles per day) for the whole population to double. For the second population it takes 2 cell cycles (n = tf = 2.6 days * 0.7692 cell cycles per day per day) for the whole population to double. Assuming N₀=100, N₁=200 for the first population and N₀=100, N₂=200 for the second population, calculate the fraction of cells dividing for each population (substitute the values you know into the general equation for fractional growth and solve for F).

Sources: Sherley, J. L., P. B. Stadler, and J. S. Stadler. 1995. A quantitative method for the analysis of mammalian cell proliferation in culture in terms of dividing and non-dividing cells. Cell Proliferation 28:137-144