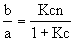
ANTIBODY BINDING
Introduction: Antibodies are proteins that react with foreign invaders during a humoral immune response. Antigens, small substituents of foreign invaders, elicit an immune response when they bind to the antibody. Variable regions of amino acid chains comprising the antibody create binding sites. A particular antibody has specificity to bind to one or more particular antigens.
Importance: Quantitatively we can describe the binding of antigens to antibodies. Experimentally, this allows us to gather information about properties of the antibody and the specificity of antibodies to particular antigens.
Questions: How is antigen binding to an antibody related to antigen concentration? How can we determine binding properties of antibodies?
Variables:
|
b |
number of bound antigens (moles) |
|
a |
total number of antibodies (moles) |
|
c |
total concentration of antigen (mols/liter) |
|
n |
number of binding sites on antibody |
|
K |
equillibrium constant of the antibody (liters/mol) |
Methods: During an immune response, the number of antibodies bound to a particular antigen depends on the concentration of that antigen as well as the number of binding sites on the antibody. Each antibody has a limited number of binding sites that become saturated as the concentration of antigen increases. This can be quantified by the following equation:
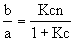
where b/a gives the proportion of bound antigens per antibody, c is the antigen concentration in the surrounding solution, and K, the equillibrium constant of the antibody, relates the speed with which the antibodies become saturated. We can plot b/a as a function of c.
Generally K and n are unknown for a particular antibody. Scatchard developed the following useful equation to describe the antigen binding by algebraically rearranging the previous equation:
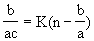
where b/ac is the number of bound antigens per antibody for a particular antigen concentration c, n is the number of binding sites on the antibody, K is the equillibrium constant of the antibody.
Experimentally, one can place antibodies in a solution of antigens and measure properties of the system at equillibrium. The system reaches equillibrium when as many antigens as possible have bound to the antibodies. At equillibrium, we can know b, a, and c, and can plot b/ac as a linear function of b/a according to the Scatchard equation. This allows us to estimate K and n for the antibody.
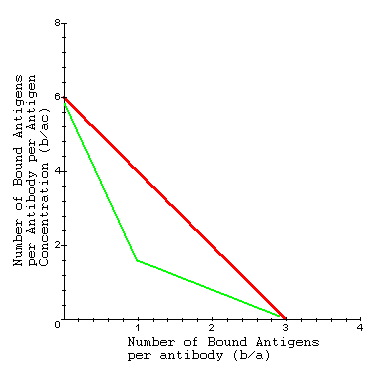
Interpretation: Using the Scatchard equation, we can then estimate the equillibrium binding constant (K) as the slope of this line (red line). A steeper slope implies the binding of antigens to antibodies reaches equillibrium more quickly (i.e. at a lower concentration or higher value of b/ac). As the antigen concentration goes to infinity we attain equillibrium. Therefore we can also estimate the number of binding sites (n) as the x-intercept (set b/ac = 0 and solve for b/a).
Experimentally, antibodies can be surrounded by a solution containing different types of antigens. When the experimental data is plotted, we do not get a straight line (green line). In this case, we can assume the antibody has specific binding sites for more than one type of antigen.
Conclusions: The binding of antigens to antibodies can be described quantitatively as a function of antigen concentration. By rearranging this equation, we can experimentally gather information about properties of the antibody.
Additional Questions:
1. Choose different values for K. How does this affect the plot of b/a as a function of c?
2. Look again at the first equation for b/a. What is b/a as the antigen concentration (c) goes to infinity?
3. Look at the line b/ac. What is the x-intercept?
Sources: Darnell, J., H. Lodish, and D. Baltimore. 1986. Molecular Cell Biology. Scientific American Books, Inc., New York
Goueli, S. A. and R. C. Steer. 1981. Some representative mathematical plots in biological research. From Advanced Cell Biology, L. M. Schwartz and M. M. Azar, eds. Litton Education Publishing, Inc., New York
Copyright 1999 M. Beals, L. Gross, S. Harrell