Introduction: Muscle fibers are composed of bundles of contractile muscle called myofibrils. Each myofibril is made up of smaller repeating units called sarcomeres. Thin filaments of actin and thick filaments of myosin form the muscle fibers. Myosin and actin filaments, as well as regions where the two overlap, form repeating light and dark bands in each sarcomere. These thick and thin filaments are linked at regular intervals by cross-bridges made from extensions of the myosin molecules.

Researchers from 1930 to 1960 sought to understand the mechanism of muscle contraction. The "contracting filament hypothesis" proposed that the filaments themselves contract. Electron microscope observations, however, did not support this hypothesis. Neither the thick nor thin bands changed in length when the muscle contracted. Only the degree of overlap between thick and thin filaments changed. Huxley alternatively propoded the Sliding Filament Model, suggesting muscle contraction results as the crossbridges linking the actin and myosin molecules pull the filaments over one another.

Importance: In a contracted muscle the degree of overlap between thick and thin filaments should be high. Consequently, Huxley hypothesized the force generated by a muscle should depend on the degree of overlap.

Question: Does evidence support the Sliding Filament Model? In other words, does the force generated by a muscle depend on the degree of overlap between thick and thin filaments?

Methods: Hill (1938) hypothesized specific relationships between the force generated by a muscle and the speed at which a stimulated muscle contracts under a given load. A stimulated muscle may contract to 1/3 its size at a particular speed. When that muscle is attached to a load, the speed and size to which it contracts decreases. In other words, as the load increases, the muscle cannot lift the load as far. Hill expressed this, for each sarcomere in a muscle, as the characteristic equation

where P describes the force generated by a muscle, V is the speed at which a muscle contracts, and a, b, c are constants. The constant a describes the force expended to make the muscle contract, and b describes the smallest contraction rate of the muscle.

We can interpret Hill's equation in the following way: as the force (P) being exerted by the muscle increases, the contraction rate (V) must decrease so that we maintain the constant, c. You can see that this makes sense by facing a friend and placing your palm flat against his. Have your friend offer resistence as you push his hand away. When your friend offers little resistance, you can rapidly displace his hand (high V) with little force (low P). When your friend offers maximal resistance, you will need maximum force (high P) to slowly (low V) displace his hand.

Hill's relationship can be rewritten as

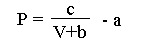
so that force (P) is now a function of the speed of contraction (V, cm/sec) of a stimulated muscle. Hill estimated that ab = g₁/f₁ where f₁ is the rate constant (attachments per second) for crossbridge connection as actin and myosin molecules combine to form crossbridges. The rate constant g₁ is the rate (detachments per second) for crossbridge detachment. The constant a is approximately equal to 1/4 P_max. P_max is the force generated in the sarcomere if all actin sites were attached to myosin sites. We can therefore simplify the above equation as

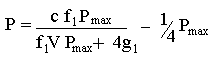
We can plot g1 versus P to predict how the force generated by a muscle depends on the rate at which crossbridges detach. From Hill's data we choose V as 2 cm/sec, f₁ as 0.21 attachments/sec, P_max as 57.4 g. wt., and c as 87.6 g wt cm/sec.

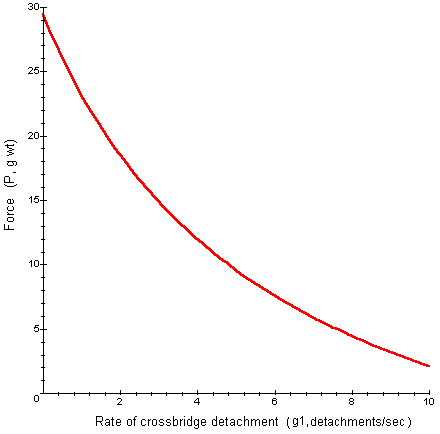
Interpretation: Notice in the graph, the force generated by a muscle is high when the rate of crossbridge detachment is low. When the rate of detachment is low, the muscle is highly contracted and there is a great deal of overlap between thick and thin filaments. As the rate of detachment increases, the degree of overlap decreases, as does the force generated by the muscle.

Huxley later derived a more precise equation based on the same principles relating force to the rate of crossbridge detachment. Both his and Hill's equations fit well to experimental data collected by numerous scientists.

Gordon, Huxley, and Julian (1966) collected data relating the degree of overlap between actin and myosin filaments to the tension in a muscle. Their data is summarized in the following graph.

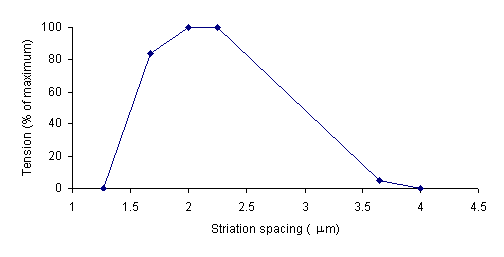
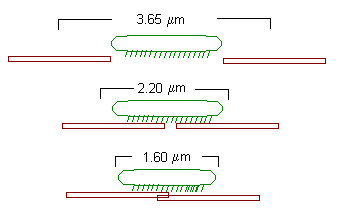
The striation spacing gives the degree of overlap. At 4 mm, the actin and myosin filaments do not overlap. Consequently the tension in the muscle is very low. As striation spacing decreases, the degree of overlap increases. Correspondingly, the degree of tension in the muscle increases. When the striation spacing is less than 2.0, the degree of overlap between actin and myosin filaments is too high for them to function optimally. Consequently, the tension in the muscle decreases.

Conclusions: The support of Hill's equation by experimental data substantiated the idea that the force generated by a muscle is related to the rate of crossbridge detachment. The research of Gordon, et al, further demonstrated that tension in muscles depends on the degree of overlap between myosin and actin filaments. Both supported Huxley's Sliding Filament Model: sarcomeres contract, and the force generated by a muscle increases, as the thick and thin filaments increase in their degree of overlap.

1. The equations of Hill and Huxley have been used recently to understand the effects of drugs on the rates of crossbridge attachment. When rabbit muscle is exposed to 2,3-butanedione monoxime, the rate constant for crossbridge attachment (f₁) decreases. How would this drug effect the graph of force (P) versus rate of crossbridge detachment (g₁)? How would you interpret such a change?

Sources: Bagni, M. A., G. Cecchi, F. Colomo, and P. Garzella. 1992. Journal of Muscle Research and Cell Motility 13:516-522.

Epstein, H. T. 1963. Elementary Biophysics: Selected Topics. Addison-Wesley Publising, Reading, MA.

Gordon, A. M., A. F. Huxley, and F. J. Julian. 1966. The variation in isometric tension with sarcomere length in vertebrate muscle fibres. Journal of Physiology 184:170-192.

Hill, A. V. 1938. The heat of shortening and the dynamic constants of muscle. Proceedings of the Royal Society of London B126:136-195.

Huxley, A. F. 1957. Muscle structure and theories of contraction. Prog. Biophysics and Biophysical Chemistry 7:255-318.